In the study of electricity and circuits, one fundamental concept that every physics or electrical engineering student encounters is resistance. Resistance plays a crucial role in determining how current flows through an electrical circuit. A particularly interesting question arises when dealing with multiple resistors: how can three resistors of resistance be combined in different ways to yield various equivalent resistances? This question is not just theoretical—it has practical applications in designing circuits, adjusting current flow, and protecting devices from overload.
This article explores in depth how can three resistors of resistance be used to achieve various configurations and values of equivalent resistance. We’ll examine the primary methods—series, parallel, and mixed combinations—supported by formulas, examples, diagrams, and applications.
Understanding Resistance and Resistors
Before diving into combinations, it’s essential to understand what resistance is.
Resistance is the opposition offered by a material or component to the flow of electric current. It is measured in ohms (Ω). A resistor is a passive electrical component with a specific resistance, used to control current in a circuit.
Three resistors, each of resistance R (or different values such as R1, R2, R3), can be combined in different ways. The goal is to understand how can three resistors of resistance be configured to yield various total or equivalent resistances.
Combining Resistors in Series
In a series combination, resistors are connected end to end, so that current flows through one resistor to get to the next. The key features of series connection are:
- The current through each resistor is the same.
- The voltage across each resistor can be different.
- Total resistance is the sum of the individual resistances.
Formula:
If three resistors R1, R2, and R3 are in series, the equivalent resistance is:
Req(series) = R1 + R2 + R3
Example:
Let’s say R1 = R2 = R3 = 5Ω
Then,
Req = 5 + 5 + 5 = 15Ω
This is the maximum resistance you can get from three resistors of equal value if connected in series.
Combining Resistors in Parallel
In a parallel combination, resistors are connected such that their terminals are directly connected to each other. Current splits among the resistors, but each one has the same voltage across it.
- The voltage across each resistor is the same.
- The total current is the sum of the currents through each resistor.
- Total resistance is less than the smallest individual resistance.
Formula:
1 / Req(parallel) = 1/R1 + 1/R2 + 1/R3
Example:
Again, using R1 = R2 = R3 = 5Ω
1 / Req = 1/5 + 1/5 + 1/5 = 3/5
So,
Req = 5 / 3 ≈ 1.67Ω
This is the minimum resistance achievable with three equal resistors.
Mixed or Series-Parallel Combination
In real circuits, it’s common to combine resistors in both series and parallel. This is especially useful for achieving intermediate equivalent resistances.
Example:
Connect R1 and R2 in parallel, and then place R3 in series with this combination.
1 / Rparallel = 1/R1 + 1/R2
Req = Rparallel + R3
If R1 = R2 = R3 = 5Ω:
1 / Rparallel = 1/5 + 1/5 = 2/5
Rparallel = 5/2 = 2.5Ω
Req = 2.5 + 5 = 7.5Ω
This configuration provides an equivalent resistance between the maximum (15Ω) and the minimum (1.67Ω).
Summary of Possible Equivalent Resistances with Three Equal Resistors (R)
If each resistor has resistance R, the various configurations can give:
| Configuration | Equivalent Resistance |
|---|---|
| All in series | 3R |
| All in parallel | R / 3 |
| Two in parallel, one in series | (2R)/(2 + 1) + R = (2R/3) + R = 5R/3 |
| Two in series, one in parallel | Req = (R + R) |
| One in series with two in parallel | R + (R |
| One in parallel with two in series | R |
So, using different arrangements, you can achieve multiple values.
Using Different Resistor Values
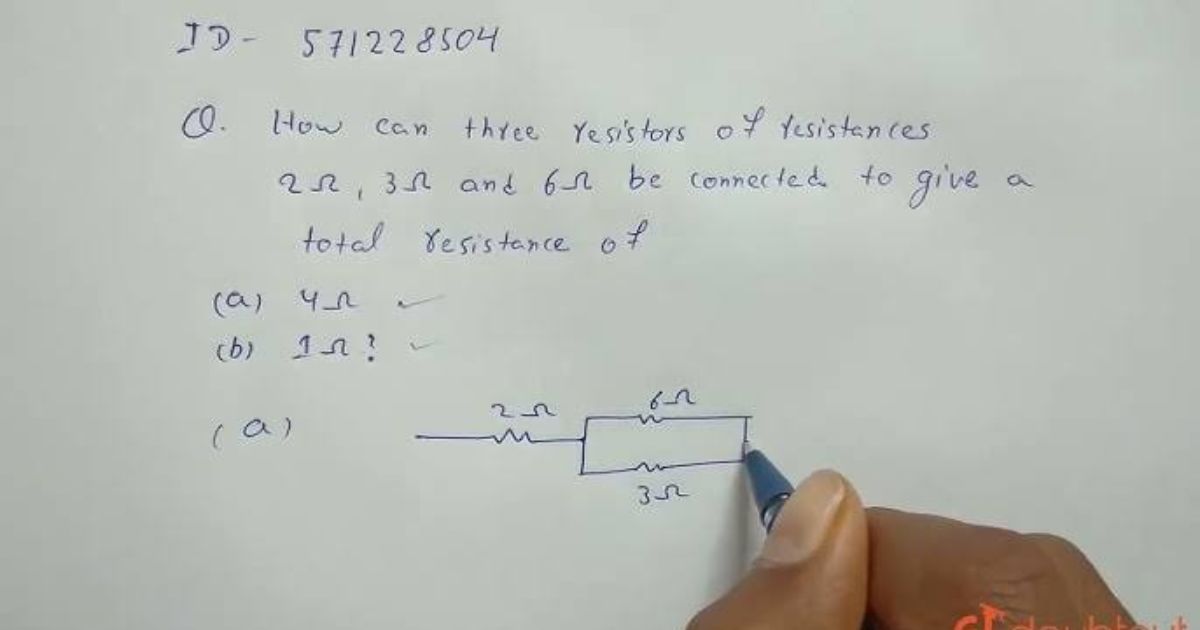
If the resistors have different values, say R1 = 2Ω, R2 = 4Ω, and R3 = 6Ω, the number of possible combinations increases. Let’s examine some scenarios:
Series:
Req = R1 + R2 + R3 = 2 + 4 + 6 = 12Ω
Parallel:
1 / Req = 1/2 + 1/4 + 1/6 = (6 + 3 + 2)/12 = 11/12
Req ≈ 1.09Ω
Series-Parallel:
- R1 and R2 in parallel: 1 / (1/2 + 1/4) = 4/3Ω
- Then in series with R3: 4/3 + 6 = 22/3 ≈ 7.33Ω
Different permutations of placement will yield different results.
Practical Applications of Resistor Combinations
Understanding how can three resistors of resistance be combined has several practical applications:
1. Voltage Division:
Series resistors divide the input voltage into parts. This is useful in sensor circuits or analog-to-digital conversion.
2. Current Limiting:
Resistors are used to protect sensitive components (like LEDs) from too much current.
3. Load Matching:
In audio electronics or radio frequency systems, specific resistance values are necessary to match impedance for maximum power transfer.
4. Creating Custom Resistance Values:
Using combinations, engineers can achieve resistance values not readily available in discrete components.
Educational Importance
This topic is a favorite in exams and lab practicals because it strengthens conceptual understanding. Students learn:
- Problem-solving skills
- Application of Ohm’s Law
- Circuit analysis techniques (e.g., Kirchhoff’s Laws)
- Use of multimeters and breadboards
Common Misconceptions
- Resistances in parallel are always smaller than the smallest resistor—this surprises many beginners.
- You can’t just add resistances in parallel—this requires reciprocal addition.
- Arrangement affects the result significantly—even with the same values, the configuration changes the outcome.
Tools to Explore Resistor Combinations
1. Online Calculators:
Web tools like All About Circuits or Digikey’s calculator can compute equivalent resistances quickly.
2. Simulation Software:
- Tinkercad Circuits
- LTSpice
- Proteus
These platforms help students and professionals visualize and simulate circuits.
3. Physical Experimentation:
Using a breadboard, three resistors, and a multimeter, anyone can experiment with combinations and verify results.
Conclusion
Understanding how can three resistors of resistance be combined is an essential skill in electronics and electrical engineering. Whether you’re designing a power supply, an audio system, or a simple LED circuit, the way resistors are arranged can significantly impact performance.
By mastering series, parallel, and hybrid configurations, one can tailor circuit behavior precisely to the requirements. With just three resistors, you can create a wide range of equivalent resistances—an impressive feat that highlights the power of foundational electrical knowledge.
Whether you’re a student, hobbyist, or professional, exploring the combinations of resistors is not only educational but also opens the door to smarter circuit design.






